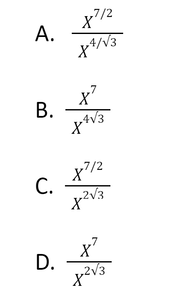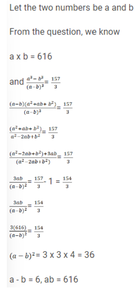01/11/2020 11:35 pm
Topic starter
Algebra
64
Posts
11
Users
107
Reactions
3,627
Views
0
1. Given the system of equations 3x + ky = 1 and 6x + 3y = 2. For which values of k does this system have infinite solutions?
32 questions & discussions are there under this sub-topic
6
19/01/2024 1:42 pm
Q1. Among 100 students, x1 have birthdays in January, x2 have birthdays in February, and so on. If x0 = max (x1, x2, ..., x12), then the smallest possible value of x0 is
Sachin Yadav 24/01/2024 4:40 pm
SOLUTION
x0 = 100 (If all 100 had their birthdays on January)
If x0 = 3 (Not possible, because all x1 ,.....x12 together will be only 36)
So x0 will be lesser when the numbers are as close as possible
100/12=8.5
So if we add 8 (12 times) = 96
So, need to have 8,8,8,8,8,8,8,8,9,9,9,9 (Adding these 12 we will get 100)
x0 = 9
5
23/12/2023 2:42 pm
Q1 If a and b are non-negative real numbers such that a+2b=6, then the average of the maximum and minimum possible values of (a+b) is
-
4
-
4.5
-
3.5
-
3
Neraj (TG) 29/12/2023 10:32 am
Answer:-
a + 2b = 6
a + b = 6 - b
Clearly, the maximum/minimum value of (a + b) depends on the value of b.
Since a and b are positive real numbers,
The maximum value that b can take is 0.
The maximum value that b can take is when a is 0.
0 + 2b = 6
b = 3.
When b = 0; a + b = 6 - b = 6
When b = 3; a + b = 6 - 3 = 3
Therefore, the minimum and maximum values of (a + b) are 3 and 6 respectively.
The average of these extreme values is (3+6)/2 = 4.5( Ans)
5
28/12/2023 5:59 pm
Q.) Consider the pair of equations: x2 - xy - x = 22 and y2 - xy + y = 34. If x > y, then x - y equals
-
8
-
6
-
7
-
4
Neraj (TG) 29/12/2023 10:26 am
ANSWER.)
x2 - xy - x= 22.
y2 - xy + y= 34.
If we add these two equations, we get
(x - y)2 - x + y = 56 or (y - x)2 - x + y = 56
Since we need to find for x > y, we should take (x - y)2 - x + y = 56
(x - y)2 - (x - y) = 56
(x - y) (x - y - 1) = 56
If we take x - y = n, then we will boil down to n (n - 1) = 56
Therefore, x - y = 8(Ans)
5
12/01/2024 4:57 pm
If f(x + 2) = f(x) + f(x + 1) for all positive integers x, and f(11) = 91, f(15) = 617, then f(10) equals.
Shivangi Garg 17/01/2024 3:40 pm
Given, f(x+2) = f(x) + f(x+1) f(11) = 91, f(15) = 617
We get 91 + f(12) = f(13)
Let f(12) be equal to some value ‘a’
So, 91 + a = f(13).
f(12) + f(13) = f(14)
a + 91 + a = f(14)
So, f(14) = 2a + 91 f(13) + f(14) = 617
So, 91 + a + 2a + 91 = 617
3a + 182 = 617
a = 145
Substituting the value of a and f(11), we get
f(10)+ 91 = 145
f(10) = 54 Hence, the answer is 54.
5
13/01/2024 7:53 pm
Q1 Veeru invested Rs 10000 at 5% simple annual interest, and exactly after two years, Joy invested Rs 8000 at 10% simple annual interest. How many years after Veeru’s investment, will their balances, i.e., principal plus accumulated interest, be equal?
Shivangi Garg 17/01/2024 3:37 pm
@neraj-naiyar Sir,
Let assume its equal after N years
Veeru’s investment after N years = 10000 + 10000 × 51005100 × N
Joy’s investment after N years = 8000 + 8000 × 1010010100 × (N – 2)
Equating both
10000 + 10000 × 51005100 × N = 8000 + 8000 × 1010010100 × (N – 2)
10000 + 500N = 8000 + 800N – 1600
3600 = 300N
N = 12
5
13/01/2024 7:54 pm
Q.5 The number of real-valued solutions of the equation 2x + 2-x = 2 - (x - 2)2 is
- infinite
- 0
- 1
- 2
Madhav 17/01/2024 4:28 pm
@neraj-naiyar Sir,
2x + 2-x is of the form y + 1/y
Minimum value the expression can take is ≥ 2
So 2x + 2-x ≥ 2
Now, 2 – (x – 2)2 ----> (x – 2)2 is ≥ 0
So 2 – (x – 2)2 ≤ 2 (From 2 we are subtracting a non-negative number)
Maximum value the expression can have is 2
The only possibility is both sides are = 2
If value = 2
Then x = 0
20 + 20 = 2 – (0 – 2)2
2 ≠ – 2
There is no case where LHS = RHS = 2
Hence 0 real-valued solutions
5
17/01/2024 3:54 pm
Q1. If log4 5 = (log4 y) (log6 √5), then y equals?
Neraj (TG) 19/01/2024 12:07 pm
Solution:-
log45 = log4y × log6√5
log45 ÷ log4y = logy5 = log6√5 = k (Let’s assume this as k)
6k = √5 ---> On squaring this {6k}2 = 5
log45 = log4y × log6√5
logy5 = log6√5
logy5 = k
yk = 5
{6k}2 = yk = 5
{62}k = yk
36k = yk
y = 36.
5
17/01/2024 4:08 pm
Q7 Let A, B and C be three positive integers such that the sum of A and the mean of B and C is 5. In addition, the sum of B and the mean of A and C is 7. Then the sum of A and B is
A. 6
B. 4
C. 7
D. 5
Neraj (TG) 19/01/2024 12:02 pm
SOLUTION
a + (b+c)/2 = 5 ---> 2a + b + c = 10
b + (a+c)/2 = 7 ---> 2b + a + c = 14
Solving these two, we get b – a = 4
b = a + 4
Substituting this in the first equation
2a + a + 4 + c = 10
3a + c = 6
Given all three as positive integers, maximum value we can get for a = 1
Ex: When substituting a = 2, c = 0 (Not possible)
So a = 1, then b = 5
Sum of a + b = 6
5
17/01/2024 4:12 pm
Q.1 If x = (4096)7+4√3, then which of the following equals 64?
Answer the Following:-?
5
19/01/2024 1:38 pm
Q1. The number of distinct real roots of the equation
(x + 1/x)2 - 3(x + 1/x) + 2 = 0 equals
Madhav 21/01/2024 7:04 pm
@neraj-naiyar Sir,
Solution:-
x + 1/x = y
y2 – 3y + 2 = 0
y ≥ 2 & y ≤ - 2
(y – 1) (y – 2) = 0
y = 1(Not possible) or y = 2
Now, x + 1/x = 2
This has only one option x = 1. So, only one real root
y2 – 3y + 2 = 0
y ≥ 2 & y ≤ - 2
(y – 1) (y – 2) = 0
y = 1(Not possible) or y = 2
Now, x + 1/x = 2
This has only one option x = 1. So, only one real root
5
24/01/2024 5:01 pm
Q1. With rectangular axes of coordinates, the number of paths from (1,1) to (8,10) via (4,6), where each step from any point (x,y) is either to (x,y+1) or to (x+1,y) is ?
Shivangi Garg 25/01/2024 11:46 am
Ans – 3920
Let us first consider travelling from (1,1) to (4, 6)
This means, Travelling from 1 to 4 units in the x axis -> 3 horizontal movements (h h h)
And travelling from 1 to 6 units in the y axis -> 5 vertical movements (v v v v v)
No matter how we proceed, reaching from (1,1) to (4,6) requires 5 vertical movements and 3 horizontal movements.
So, Number of paths to travel from (1,1) to (4,6) = Number of ways of arranging (h h h v v v v v)
5
25/01/2024 12:24 pm
Q1. A club has 256 members of whom 144 can play football, 123 can play tennis, and 132 can play cricket. Moreover, 58 members can play both football and tennis, 25 can play both cricket and tennis, while 63 can play both football and cricket. If every member can play at least one game, then the number of members who can play only tennis is ?
- 32
- 43
- 38
- 45
Neraj (TG) 27/01/2024 12:52 pm
Ans – 43
From observing the data given, we find that it is a closed 3 set Venn diagram.
Let the three sports be F, T and C for Football, Tennis and Cricket respectively
n(FUTUC) = 256, n(F) = 144, n(T) = 123, n(C) = 132, n(F T) = 58, n(C ∩T) = 25, n(F C) = 63
5
27/01/2024 1:20 pm
Q1. For any positive integer n, let f(n) = n(n + 1) if n is even, and f(n) = n + 3 if n is odd. If m is a positive integer such that 8f(m + 1) - f(m) = 2, then m equals
Neraj (TG) 28/01/2024 6:00 pm
Ans – 10
If n is even, f(n) = n (n + 1)
So, f (2) = 2(2+1) = 2 (3) = 6
If n is odd, f(n) = n + 3
f (1) = 1 + 3 = 4
It is given that, 8 x f(m+1) - f(m) =2
So, m can either be even or odd
Case-1: If m were even and m+1 odd
So, 8 x f(m+1) - f(m) =2
8(m + 4) - m (m + 1) = 2
8m + 32 - m2 - m = 2
m2 - 7m - 30 = 0
(m-10) (m+3) = 0
m = 10 or -3
m = 10, since m is positive
Case-2: If m were even and m+1 odd
8 x f(m+1) - f(m) =2
8 (m +1) (m + 2) - (m + 3) = 2
Now, let us substitute m = 1 which is the minimum possible value
8 (1 + 1) (1 + 2) - (1 + 3) 3
Case 2 does not work.
5
28/01/2024 6:56 pm
Q1. The product of two positive numbers is 616. If the ratio of the difference of their cubes to the cube of their difference is 157 : 3, then the sum of the two numbers is
Shivangi Garg 29/01/2024 2:28 pm
@neraj-naiyar Sir,
Ans - 50
616 can be rewritten as 22 x 28, where 28 - 22= 6 using trial and error
So, a + b = 28 + 22 = 50
Page 1 / 3
Next