27/10/2018 11:28 am
Algebra
297
Posts
26
Users
18
Reactions
56.6 K
Views
0
Find the largest natural number which is divisible by all the numbers less than or equal to its square root?
16
36
24
aniket prajapati 27/10/2018 2:14 pm
24 is the answer its under root will b around 4.9 and natural no.s less than 4.9 are 4,3,2,1 and all are divisible by 24
0
27/10/2018 5:55 pm
I have got it as
8^3-1/8^3+1 ...... And so on but what to do next ?
0
29/10/2018 10:15 am
How many three digit numbers of base-10 both start and end with 1 when represented in base-3, base-5 or base-7 representations?
0
29/10/2018 10:18 am
Find the largest number that is divisible by all of positive integers less than it's cuberoot.
aniket prajapati 29/10/2018 2:27 pm
One way is to check the options if options are not given then only way is to fiddle with nos...
0
29/10/2018 2:30 pm
Find minimum value of a + b + c if a, b, c are distinct positive integers such that 1/a + 1/b + 1/c = 1.
6
7
9
aniket prajapati 29/10/2018 5:53 pm
By A.M >= H.M
we have three nos. i.e a,b,c
(a+b+c)/3 >= 3/{(1/a)+(1/b)+(1/c)}
Since (1/a)+(1/b)+(1/c) =1
a+b+c>=9
So the minimum Value is 9
0
29/10/2018 2:36 pm
In a regular polygon of 2012 sides, distance from centre of polygon to a vertex is 5cm. Find the area of the polygon approximately.
44
31
78
aniket prajapati 29/10/2018 5:58 pm
If we find out interior angle it will be
(n-2)180/n where n is the no. Of sides i.e 2012
2010*180/2012 approx value will be 180 so it will be almost a circle
Area = π×5*5 = 22*25/7
= 78.5 hence option third
0
29/10/2018 6:14 pm
Three men make the following statements regarding a murder that they are suspected of. Two of the men are lying, and one of them is telling the truth. Only one of the men committed the crime.
A says: I didn’t do it.
B says: C did it.
C says: A did it.
Who is certainly lying?
A
B
C
CBD
Which of the three is most likely to be innocent?
A
B
C
CBD
This post was modified 6 years ago by Utkarsh Garg
aniket prajapati 29/10/2018 7:09 pm
We have to take three cases
Case 1 if a says truth
Since a is saying true so he didn't do it, it would be b or c
B is lying so c didnt do it
And c is lying so a didnt do it which was already told by a so b is the criminal
Case 2 if b is saying true
Since a is saying false means he did it
B said c did it which is true so that means c did it since question says only one has done the crime so this case isnt true
3rd case if c is saying true
A is lying so he did it
B is lying so c didnt do it and C is saying true which we got earlier by A
So in all three cases b is certainly lying and c is most likely to be innocent
0
29/10/2018 8:27 pm
Keshav and Krishna start a game with one pile of 40 coins. They take turns. On each turn, a player must take 1, 3, or 4 coins from the pile. The player, who takes the last coin from the pile of 40 coins, wins the game.
If Keshav plays first, what number of coins must he take from the pile on his first turn in order to guarantee that he can win the game?
1
3
4
Keshav can never win
If Krishna starts the game by taking 2 coins from pile, then who has the winning strategy?
Krishna
Keshav
No one has winning strategy
data insufficient
0
29/10/2018 9:08 pm
hi sir, please help
aniket prajapati 29/10/2018 10:49 pm
Puttning x=0 and y=402 we have
f(402)= f(f(402))
f-1(f(402))=f(402)
since f-1(f(x)) =x { property}
402=f(402)
option 3rd
0
30/10/2018 1:56 am
Please tell the approach
Ans- option (A)
0
30/10/2018 1:57 am
aniket prajapati 30/10/2018 8:23 am
Let roots be p,q,r
p+q+r= a.........(1)
pq+qr+pr=b
pqr=a …..........(2)
If there are three no. p,q,r
By A.M>= G.M
(p+q+r)/3>=(pqr)^(1/3)
Putting value from 1 and 2
a^3/27>=a
a^2 >=27
Hence a can't be equal to 0 is the answer
TG Team 30/10/2018 3:47 pm
x³ - ax² + bx -a = 0
x² ( x - a) + b ( x-a) = 0
if , b = 1 , then the equation reduces to
x²( x - a) + (x-a) = 0
(x-a)(x² + 1) = 0
it has one real root a and two imaginary roots . hence b ≠ 1 .
Option D.
0
30/10/2018 11:34 am
Que.10
In annual dealer meet of Lam Organics, all 101 dealers are gathered and they shook hands with each other. It was known later that each dealer shook hands with at least 68 other dealers. Find the minimum number of dealers who shook hands with same number of other dealers.
0
2
3
4
0
30/10/2018 11:36 am
Que.12
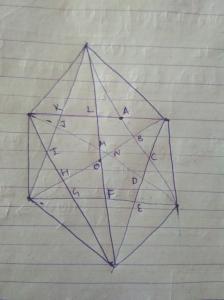
Diagonals of a quadrilateral intersect in 1 point, and that of a pentagon intersect in at most 5 distinct points. What is the maximum number of points of intersections of diagonals in a hexagon?
6
9
10
15
0
30/10/2018 11:36 am
Find the number of integral triplets (a, b, c) such that 1 ≤ a ≤ b ≤ c ≤ 10.
120
180
220
286
TG Team 30/10/2018 12:46 pm
All distinct a < b < c .
10C3 => 120
two same 10C1 x 9C1 => 90 cases
a=b = c
10 cases
Total : 120 + 90 + 10 = 220.
0
30/10/2018 11:36 am
What are the last two digits of 9119 – 1991?
62
38
82
18
TG Team 30/10/2018 3:34 pm
last two digits of 91^19 => 11
last two digits of 19^91 => 19
last two digits of 91^19 - 19^91 => 08
options are wrong.