19/02/2018 6:39 pm
Topic starter
0
India is a multi-religion, multi-language and multi-cultural country
where people belonging to different religions join in celebrating
the festivities together. The Indian Government declares such big
occasions as public holidays to enable the citizens to enjoy and
foster the feelings of brotherhood.Five broad-minded persons
belonging to different religions were asked to give their preferences
of four such festivals which they would like to enjoy with likeminded
brethren. Their options are
A. Holi, Dussehra, Diwali, Guru Nanak Birthday
B. Shivratri, Christmas, Onam, Eid
C. Holi, Shivratri, Christmas, Diwali
D. Holi, Dussehra, Guru Nanak Birthday, Eid
E. Christmas, Diwali, Onam, Guru Nanak Birthday
67. Which pair celebrates Christmas and Onam but not Dussehra
and Holi?
(a) A and C (b) A and E
(c) B and D (d) B and E
68. Which pair does not participate in Eid and Onam but joins in
Holi?
(a) A and B (b) A and C
(c) C and D (d) D and E
69. Who enjoys Holi and Eid but not Diwali and Christmas?
(a) B (b) C
(c) D (d) E
70. Which of the following expressions will be true if the
expression R > O = A > S < T as definitely true?
(a) O > T (b) S < R
(c) T < A (d) S = O
17 questions & discussions are there under this sub-topic
1
20/07/2018 9:18 am
TG Team 20/07/2018 3:33 pm
Hello Apoorva!
From the first statement :
B < C , D < A , C < E
From the second statement :
A > F , A > G , F > D
and G > F .
now , G was as old as B when A was as old as G .
Hence , A = B .
Combining the results we get : E > C > B = A > G > F > D .
10. (B) E
11. (B) D
12. (C) A and B
13. (C)
1
31/08/2018 2:09 pm
0
02/08/2018 3:12 pm
Sir please help me with this set.
0
31/08/2018 2:09 pm
0
28/09/2018 7:47 pm
0
30/09/2018 9:08 pm
I found this solution
0
03/10/2018 5:38 pm
TG Team 04/10/2018 12:45 pm
Let cheque amount be Rs X and Y paise.
The confused bank teller cashed Rs Y and X paise.
It is given that after buying a toffee for 50 paise, Shailaja is left with 3 times of the amount on the cheque.
Therefore, 3(X + Y/100) = (Y + X/100 – 50/100)
(299X + 50)/100 = (97Y/100)
Y = 3X + (8X + 50)/97
X = 18 gives an integral value of Y.
Hence Option (D)
0
10/10/2018 7:04 pm
Utkarsh Garg 10/10/2018 7:58 pm
This post was modified 6 years ago by Utkarsh Garg
Its very Time consuming..
You have to consider all the possibilities to solve this set.
0
19/10/2018 8:29 pm
What should be the answer? And please attach the solution.
aniket prajapati 19/10/2018 9:16 pm
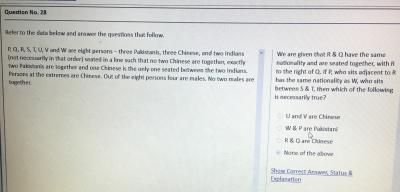
P=pakistan
C=chinese
I=indian
C P P I C I P C
Q R P S/T W S/T
we are left with U and V which will occupy C place hence option A
0
20/10/2018 8:06 am
Q6
TG Team 20/10/2018 10:16 am
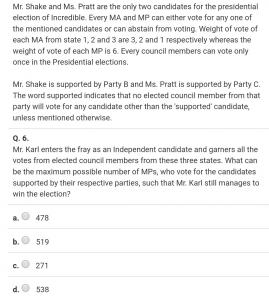
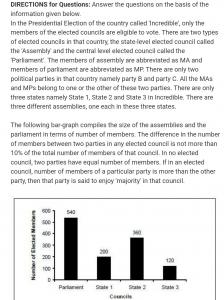
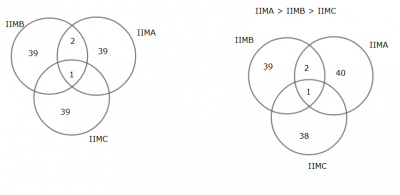
To find minimum number of students who attended admission process of IIMA , which is the maximum ( IIMA > IIMB > IIMC ) , assign minimum values for those who attended admission process of all the three IIMs and those who attended exactly two and distribute the remaining equally :
Minimum: 40 + 2 + 3 = 43
0
23/10/2018 5:19 pm
0
14/11/2018 5:34 pm
0
30/06/2019 9:33 pm
Gaurav Jain 02/07/2019 4:27 pm
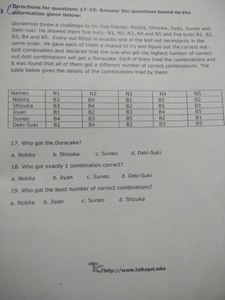
Correct answer combinations can be 5,4,3,2,1
or 5,3,2,1,0
when we check through 5,4,3,2,1..Then there should be atleast 2 people with 4 same answers. But there are no such 2 people
Hence correct answer combination will be 5 ,3 ,2 ,1, 0
now there there are 3 matches for nobita and shizuka, hence one of them answered 4 correctly.
Further we check then there is only one person who has 2 matches with shizuka and there is no such person for nobita, hence shizuka answered 4 of them correctly.
Now with shizuka's answer we can answer all other questions
Page 1 / 2
Next