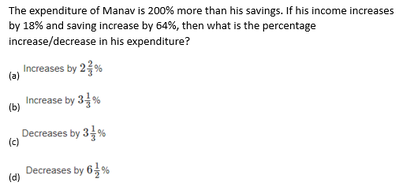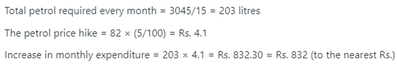04/08/2024 5:32 pm
Percentage and CI SI
111
Posts
27
Users
22
Reactions
26.7 K
Views
0
Saurabh spends 20% of his monthly income on the education of his children. He spends 30% of the remaining on convenience and clothes. Further, he spends 50% of the amount still with him on rent and maintenance of the house. After all the expenditure, he sends 50% of the amount still with him to his parents. He saves the remaining Rs. 11,200.
What is his monthly income?
- 86,000
- 11,200
- 1,00,000
- 80,000
Neraj (TG) 04/08/2024 7:03 pm
Let the entire salary = 100%
Education expenditure = 20%
Conveyance and clothes expenditure = (100 - 20) × 30% = 24%
Rent and maintenance of the house = (100 – 20 - 24) × 50% = 28%
Remaining = 100 – 20 – 24 – 28 = 28%
Percentage sent to his parents = 28 × 50% = 14%
Remaining with him = 28 – 14 = 14%
According to question,
14% of the income = 11200
So, his total income = 80,000
0
04/08/2024 5:38 pm
In a school, 4% of the students did not appear for the annual exams. 10% of the students who appeared for the exams could not pass the exam. Out of the remaining students, 50% got distinction marks and 432 students passed the exam but could not get distinction marks. The total number of students in the school is:
- 878
- 960
- 1200
- 1000
Neraj (TG) 04/08/2024 7:02 pm
Let total number of students be 100x
Number of students who did not appear = 100x x (4/100) = 4x
Number of appeared students = 100x-4x = 96x
Number of appeared students who could not pass = 96x x (10/100) = 9.6x
Remaining students who passed = 96x-9.6x = 86.4x
Number of students who only passed but couldn't get distinction marks = 86.4x x 1/2 = 43.2x
Now, 43.2x = 432
x = 432/43.2
⇒ x = 10
... Total number of students = 100 × 10 = 1000
0
04/08/2024 5:54 pm
Three friends A, B, and C started a business, each investing Rs. 10,000. After 5 months A withdrew Rs. 3000, B withdrew Rs. 2000 and C invested Rs. 3000 more. At the end of the year, a total profit of Rs. 34,600 was recorded. Find the share of each.
Neraj (TG) 04/08/2024 7:01 pm
We know that if the period of investment is not uniform, the gains/losses from the business are divided in the ratio of their inputs, where input is calculated as the product of an amount of investment and the time period of investment.
So, input = value of investment x period of investment, and here, the period of investment would be broken into parts as the investment is not uniform throughout the time period.
A’s input = (10,000 x 5) + (7,000 x 7) = 99,000
B’s input = (10,000 x 5) + (8,000 x 7) = 1,06,000
C’s input = (10,000 x 5) + (13,000 x 7) = 1,41,000
=> A : B : C = 99000 : 106000 : 141000
=> A : B : C = 99 : 106 : 141
=> A : B : C = (99 / 346) : (106 / 346) : (141 / 346)
Thus, A’s share = (99 / 346) x 34600 = Rs. 9900
B’s share = (106 / 346) x 34600 = Rs. 10600
C’s share = (141 / 346) x 34600 = Rs. 14100
0
16/08/2024 6:56 pm
The population of a town increased by 12% in the first year and by 16% in the second year. What is the percentage increase in the population at the end of the second year with respect to the population at the beginning of the given 2- year period?
(a) 29.6%
(b) 29.92%
(c) 19.92%
(d) 19.6%
Neraj (TG) 16/08/2024 7:02 pm
The population increased in 1st year = 100 + 12 = 112
New population = 112
The population increased in 2nd year = 112 × 16% of 112 = 129.92
Total percentage population increased in 2 years is 29.92%
0
16/08/2024 6:58 pm
A candidate got 41250 votes in an election and defeated his opponent by a margin of 7500 votes. If no votes were declared invalid and there were only two candidates, then what is the percentage of votes obtained by the winning candidate?
(a) 65
(b) 55
(c) 60
(d) 75
0
22/08/2024 6:52 pm
A candidate got 41250 votes in an election and defeated his opponent by a margin of 7500 votes. If no votes were declared invalid and there were only two candidates, then what is the percentage of votes obtained by the winning candidate?
(a) 65
(b) 55
(c) 60
(d) 75
0
22/08/2024 6:52 pm
Saurabh spends 20% of his monthly income on the education of his children. He spends 30% of the remaining on coveyance and clothes. Further, he spends 50% of the amount still with him on rent and maintenance of the house. After all the expenditure, he sends 50% of the amount still with him to his parents. He saves the remaining Rs. 11,200.
What is his monthly income?
- 86,000
- 11,200
- 1,00,000
- 80,000
Neraj (TG) 22/08/2024 6:55 pm
Let the entire salary = 100%
Education expenditure = 20%
Conveyance and clothes expenditure = (100 - 20) × 30% = 24%
Rent and maintenance of the house = (100 – 20 - 24) × 50% = 28%
Remaining = 100 – 20 – 24 – 28 = 28%
Percentage sent to his parents = 28 × 50% = 14%
Remaining with him = 28 – 14 = 14%
According to question,
14% of the income = 11200
So, his total income = 80,000
0
22/08/2024 6:52 pm
A dairy owner sells 528 litres of milk daily in one litre packets of 3 varieties - full cream, toned and double toned. Sales of toned milk packets are 80% higher than those of full cream milk packets, and sales of double toned milk packets are 60% lower than those of toned milk packets. How many packets of double toned milk does he sell per day?
(a) 100
(b) 112
(c) 108
(d) 110
Neraj (TG) 22/08/2024 6:55 pm
Let Q packet of full cream milk be sold.
Number of packets of toned milk sold = Q + Q × 80%
⇒ 1.8Q
Number of packets of double toned milk sold = 1.8Q-1.8 × 60%
⇒ 0.72Q
According to the question, Q+1.8Q+0.72Q = 528
3.52Q = 528
Q=528/3.52
⇒ Q = 150
Now, number of double toned milk per day = 150 × 0.72 = 108
0
22/08/2024 6:53 pm
The price of petrol shot up by 5%. Before the hike, the price was Rs. 82 per litre. A man travels 3045 km every month and his car gives a mileage of 15 km per litre. What is the increase in the monthly expenditure (to the nearest Rs.) on the man's travel due to the hike in the petrol prices?
- 944
- 859
- 758
- 832
0
22/08/2024 6:53 pm
The price of petrol shot up by 5%. Before the hike, the price was Rs. 82 per litre. A man travels 3045 km every month and his car gives a mileage of 15 km per litre. What is the increase in the monthly expenditure (to the nearest Rs.) on the man's travel due to the hike in the petrol prices?
- 944
- 859
- 758
- 832
0
22/08/2024 6:54 pm
In an examination, B obtained 20% more marks than those obtained by A, and A obtained 10% less marks than those obtained by C. D obtained 20% more marks than those obtained by C. By what percentage are the marks obtained by D more than those obtained by A?
- 33.33%
- 13.33%
- 43.33%
- 23.33%
Neraj (TG) 22/08/2024 6:56 pm
Let the marks obtained by C be 100
D obtained 20% more marks than those obtained by C
(120/100) x 100 = 120
A obtained 10% less marks than those obtained by C
(90/100) x 100 = 90
B obtained 20% more marks than those obtained by A
⇒(120/100) x 90 = 108
Now, The percentage of marks obtained by D more than those obtained by A = [(120-90)/90 x 100]
(30/90) x 100 = 100/3% = 33.33%
Page 4 / 4
Prev