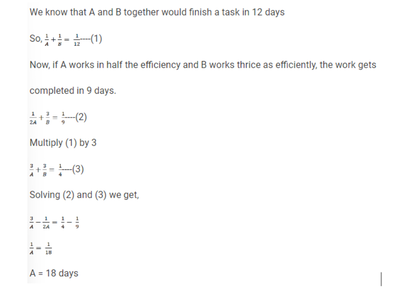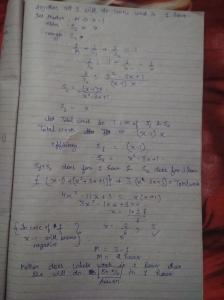20/02/2018 5:50 pm
Topic starter
Ratio - proportion, Time and work
42
Posts
18
Users
21
Reactions
17.4 K
Views
1
A works twice as fast as B. If B can complete a work in 18 days independently, the number of days in which A and B can together finish the work in:
(a.) 2 days
(b.) 6 days
(c.) 4 days
(d.) 5 days
(e.) None of thes
24 questions & discussions are there under this sub-topic
5
23/12/2023 2:38 pm
Q1 Working alone, the times taken by Anu, Tanu and Manu to complete any job are in the ratio 5 : 8 : 10. They accept a job which they can finish in 4 days if they all work together for 8 hours per day. However, Anu and Tanu work together for the first 6 days, working 6 hours 40 minutes per day. Then, the number of hours that Manu will take to complete the remaining job working alone is?
Neraj (TG) 24/12/2023 3:19 pm
Time taken by Anu, Tanu and Manu to complete a job is in the ratio 5 : 8 : 10
This means that their efficiencies are in the ratio 1/5:1/8:1/10
Or, their efficiencies are in the ratios 8 : 5 : 4
This means, for instance, if Anu can wash 8 plates in an hour, Tanu and Manu can wash 5 & 4 plates respectively in one hour.
So, let us remodel the entire question over this imaginary scenario of washing plates…
All three of them finish the job in 4 days working 8 hours per day.
Anu, Tanu and Manu can wash 8, 5 and 4 plates respectively in an hour.
So the total number of plates = (8+5+4)×4×8 = 17×4×8
Anu and Tanu work together for 6 days, working 6 hours 40 minutes per day.
The total number of plates washed by them = (8+5)×6×20/3 = 13×40
So the remaining plates to be washed =17×4×8−13×40
=4(17×8−130)
=4(80+56−130)
=4×6
So, the question is, in how many hours can Manu wash 4×6 plates?
We know that Manu can wash 4 plates in one hour.
Therefore, he requires 6 hours to wash 4×6 plates.
The answer is '6'
5
24/12/2023 3:58 pm
Q1.)A group of N people worked on a project. They finished 35% of the project by working 7 hours a day for 10 days. Thereafter, 10 people left the group and the remaining people finished the rest of the project in 14 days by working 10 hours a day. Then the value of N is
A. 23
B. 140
C. 36
D. 150
This post was modified 12 months ago by Sachin Yadav
Neraj (TG) 26/12/2023 11:29 am
Answer :-
N people finish 35% of the project by working 7 hours a day for 10 days.
N people finish 35% of the project by working 70 hours.
N people finish 5% of the project by working 10 hours.
N people finish 65% of the project by working 130 hours.
65% of the project is done in N * 130 man hours.
The remaining 65% was actually done by (N-10) people in 14 days by working 10 hours a day.
(N-10) people finish 65% of the project by working 140 hours.
65% of the project is done in (N - 10) * 140 man hours.
N * 130 = (N - 10) * 140
13N = 14N - 140
N = 140
3
12/01/2024 4:59 pm
When they work alone, B needs 25% more time to finish a job than A does. They two finish the job in 13 days in the following manner: A works alone till half the job is done, then A and B work together for four days, and finally B works alone to complete the remaining 5% of the job. In how many days can B alone finish the entire job?
Ujjwal Dwivedi 17/01/2024 3:43 pm
Given, f(x+2) = f(x) + f(x+1) f(11) = 91, f(15) = 617
We get 91 + f(12) = f(13)
Let f(12) be equal to some value ‘a’
So, 91 + a = f(13).
f(12) + f(13) = f(14)
a + 91 + a = f(14)
So, f(14) = 2a + 91 f(13) + f(14) = 617
So, 91 + a + 2a + 91 = 617
3a + 182 = 617
a = 145
Substituting the value of a and f(11), we get
f(10)+ 91 = 145
f(10) = 54 Hence, the answer is 54.
3
30/01/2024 3:32 pm
Q1. At their usual efficiency levels, A and B together finish a task in 12 days. If A had worked half as efficiently as she usually does, and B had worked thrice as efficiently as he usually does, the task would have been completed in 9 days. How many days would A take to finish the task if she works alone at her usual efficiency?
1
16/03/2018 10:21 am
Well done Vidisha, continue to ask questions
Let total work = 120 unit
And the work completed after n days.
1st day work =1 unit
2n day work = 2 unit
3rd day work = 3 unit
………………..
………………..
nth day work = n unit
1 + 2 + 3+ 4 + …………….+n =120
n (n+1)/2 = 120
n2 + n = 240
n2 + n – 240 = 0
n2 + 16n -15n – 240 =0
n(n+ 16) – 15(n + 16) = 0
(n-15)(n+ 16) = 0
n = 15 or n =-16
Required days = 15
1
05/01/2019 6:24 pm
A and B together can do a piece of work in 7 days. If A does twice as much work as vinay in a givem timw, how long will arun alonw take to do the work?
TG Team 05/01/2019 6:41 pm
Let’s assume
Time taken by A to do the work : x days
so time taken by B: 2x days
work done by A in 1 day : 1/x units
Work done by B in 1 day : 1/2x units
Work done by A and B in 1 day = 1/7 units
So, 1/x + 1/2x = 1/7
Solving this we get x = 21/2
So A alone takes 21/2 days .
1
05/01/2019 6:41 pm
Raju can do a work in 10 days, vicky in 12 days and tinku in 15 days. They all started the work together, but raju leaves after 2 days and vicky leaves 3 days before the work is completed. In how many days is the work completed?
TG Team 05/01/2019 7:05 pm
Total work : 60 units [ LCM of 10,12 and 15]
Raju can do the work in 10 days so he does 60/10 = 6 units / day
similarly Vicky does 5 units/ day and
Tinku does 4 units per day
Raju left the work after 2 days so work done by Raju , Vicky and Tinku in first two days = ( 6 + 5 + 4) x 2 = 30 units
Vicky left the work 3 days before its completion , so Tinku alone does : 4 x 3 = 12 units
So remaining ( 60 - 30 - 12) = 18 units work was done by Vicky and Tinku so 18/ ( 4+5) = 2 days
So total days : 2 + 2 + 3 = 7 days.
0
22/02/2018 12:37 pm
Hello Tina,
Please find the solution:
Let total work is 18 unit.
B can complete 1 unit work in 1 day.
So, A can complete 2 unit work in 1 day.
One day work of A and B = 1 + 2 = 3 unit.
Required days to complete 18 unit by A and B both = 18/3 = 6 days.
The correct Answer is B.
0
15/03/2018 11:15 am
Hello sir,
Please solved this question. This question was asked in Cat 2017 in slot- 1
A person can complete a job in 120 days. He works alone on Day 1. On Day 2, he is joined by another person who also can complete the job in exactly 120 days. On Day 3, they are joined by another person of equal efficiency. Like this, every day a new person with the same efficiency joins the work. How many days are required to complete the job?
0
31/03/2018 2:57 pm
The efficiency of man is reduced by half every two hours.He could have completed the work in 150 hours at maximum efficiency.How many hours does he take if his efficiency becomes maximum after every 8 hours and then reduces as mentioned above?
0
31/03/2018 2:57 pm
Hello Vidisha,
Please find the solution below.
At maximum efficiency, he finishes the work in 150 hrs that means the amount of work done in 2 hrs at maximum efficiency = 1/75.
For next two hours he finishes = 1/150 amount of work.
In next two hours he does = 1/300 amount of work and
in another two hours, he finishes = 1/600 amount of work.
That means in total 8 hrs he does = (1/75)(1 + 0.5 + 0.25 + 0.125) = 1.875/75 = 1/40 amount of work.
As he regains his maximum efficiency after every 8 hrs, he does the same amount of work in every 8 hrs span.
So total time required to complete the work = 8*40 = 320 hrs.
0
07/08/2018 2:05 pm
A worker working under a bonus scheme saves 10 hours in a job for which the standard time is 60 hrs. A bonus of 10% of the hourly rate is payable when he reaches 100% efficiency. An additional bonus of 2% of the hourly rate for each 1% in excess of 100% efficiency is given. If the normal wage rate is Rs.2 per hour, find the wages payable to the worker.
0
24/09/2018 1:12 am
A mother along with her two sons is entrusted with the task of cooking biryani for a family get together. It takes 30 minutes for all three of them cooking together to complete 50 percent of the task. The cooking can also be completed if the two sons start cooking together and the elder son leaves after 1 hour and the younger son cooks for further 3 hours. If the mother needs 1 hour less than the elder son to complete the cooking, how much cooking does the mother complete in an hour?
a. 33.33%
b. 50%
c. 66.67%
d. none of these
0
23/10/2018 2:42 pm
solution please
TG Team 23/10/2018 3:06 pm
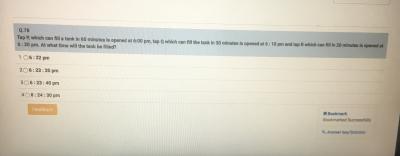
Let the time in which tank will be filled is x minutes
So,
x/60 + ( x -10)/30 + ( x-20)/20 = 1
on solving x = 70/3 i.e 23 minutes 20 seconds
Hence , option 2.
Page 1 / 2
Next