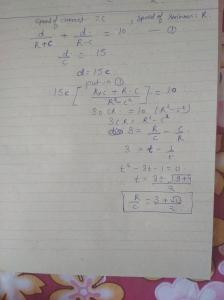20/02/2018 2:41 pm
Topic starter
0
Hemant and Ajay start a two-length swimming race at the same moment but from opposite ends of the pool. They swim in lane and at uniform speed, but Hemant is faster than Ajay.They first pass at a point 18.5 m from the deep end and having completed one length, each one is allowed to rest on the edge for exactly 45 seconds.After setting off on the return length, the swimmers pass for the second time just 10.5 m from the shallow end.How long is the pool?
Options:
a.55.5m, b.45m, c.66m, d.49m.
Anyone please explain the procedure to solve the above problems.Thanks in advance.
8 questions & discussions are there under this sub-topic
1
22/02/2018 11:44 am
Hello Kinshuk,
Let the length of the pool be d, speed of Hemant and Ajay be h and a.
When the meet for the first time, they have travelled a distance of d while when the meet for the second time, they have travelled a distance of 3d together.
Thus the distance travelled by a for both the meetings, would be in the ratio 1:3.
Thus (d+10.5)/18.5=3 => d=45m
0
09/07/2018 1:59 am
Raju takes 4 hours less to row down a 12 km stream than he takes to row up, for this 24 km round trip, if he doubles his rowing speed, he would take half an hour less to row downstream than to row upstream. Find the speed of the stream in km/hr?
tothepoint 16/07/2019 10:12 am
Assume B = Speed of a boat in still water, and C = Speed of current.
12/(B-C) - 4 = 12/(B+C)
and
12/(2B-C) - 1/2 = 12/(2B+C)
Solving, this, we get C = 8 and B = 4*sqrt7.
0
11/08/2018 6:38 pm
0
08/10/2018 1:49 pm
A man wishes to cross a river perpendicularly. In still water he takes 4 minutes to cross the river , but in flowing river he takes 5 minutes. If the river is 100 meters wide , the velocity of the flowing water of the river is?
aniket prajapati 08/10/2018 9:42 pm
speed of man =100/(4*60) m/s
=5/12
ratio of time of man alone and man flowing in water is 4:5
ratio of speed =5:4
5x=5/12
x=1/12
speed of flowing water=5x-4x=x
x=1/12 m/s (answer)
aniket prajapati 09/10/2018 6:37 pm
This post was modified 6 years ago by aniket prajapati
Hi Nikita, the water will apply force perpendicularly so instead of swimming 100 m he will swim 125 m in 5 min so given right angled traingle will be formed and water will cover 75 m in the same time.
0
08/11/2018 11:43 am
Two kayak racing champions Santa and Banta challenge each other to a 12 km downstream kayak race. Santa rows downstream at 6kmph whereas Banta rows the same stretch at 4 kmph. They start at the same time from the same place but at one point, Santa loses his paddle and if forced to complete the remaining part of his race at half the initial speed. If he loses the race by 12 mins, how many kms from the end did he lose the paddle?
3
4
4.8
aniket prajapati 08/11/2018 2:01 pm
let after t1 time santa loses his paddle then after t1 time distances covered by them is 6t1 and 4t1
after this banta takes t2 time to get to the end and santa takes (t2- 12/60)= (t2- 1/5)
total distance is 12 so we have two equation
6t1 + 3(t2-1/5) = 12 { 3 becuase after time t1 santa rows at half of the initial speed}
for banta
4t1+ 4t2= 12
solving these two we get t1 = 1.2
so before he lost his paddle he rowed = 6*1.2= 7.2
distance from the end of the race =12-7.2 =4.8
0
01/05/2024 11:40 am
(a) 4 (b) 4.5 (c) 5 (d) 5.5