20/02/2018 2:42 pm
Topic starter
TSD (Time speed and distance)
142
Posts
34
Users
43
Reactions
46 K
Views
0
Two cars (A and B) start at the starting line at the same time on a 3-mile long circular track, going in opposite directions. As they drive around the course, they pass each other many times. Exactly one hour after starting, they pass each other for the 33rd time. At this point car A has completed exactly 20 laps. What is the average speed of Car B?
65 questions & discussions are there under this sub-topic
5
22/12/2023 3:16 pm
Q.
Trains A and B start traveling at the same time towards each other with constant speeds from stations X and Y, respectively. Train A reaches station Y in 10 minutes while train B takes 9 minutes to reach station X after meeting train A. Then the total time taken, in minutes, by train B to travel from station Y to station X is
15
12
6
10
Shivangi Garg 23/12/2023 5:53 pm
Let the meeting point of the two trains between stations X and Y be M.
Let the time taken to reach M from X by train A be ‘t’ minutes. Since the two trains start simultaneously, the time taken by train B to reach M from Y will also be ‘t’ minutes.
We know that train A completes the entire journey in 10 minutes, so the time taken by train A to travel from M to Y will be ‘10 - t’ minutes. We are told that train B takes 9 minutes to reach X after it meets train A, which means, train B takes 9 minutes to travel from M to X.
When two bodies travel equal distances at constant speeds, the ratio of time taken by them to travel those distances will be the same.
The time taken by A and B to travel the distance between A and M is ‘t’ minutes and 9 minutes respectively. Similarly, the time taken by them to travel the distance between M and B is ‘10 - t’ and ‘t’ minutes.
The ratio of the times taken should be equal.
t/9=(10−t)/t
=9(10−t)
=90−9t
+9t−90=0
+15t−6t−90=0
t(t+15)−6(t+15)=0
(t+15)(t−6)=0
t = - 15 or t = 6
t can’t be negative, therefore, t is 6.
The total time taken by train B to reach station X from station Y is 6 + 9 =
15 minutes.
THE ANSWER IS 15 MINUTES.
Shivangi Garg 23/12/2023 5:54 pm
Let the meeting point of the two trains between stations X and Y be M.
Let the time taken to reach M from X by train A be ‘t’ minutes. Since the two trains start simultaneously, the time taken by train B to reach M from Y will also be ‘t’ minutes.
We know that train A completes the entire journey in 10 minutes, so the time taken by train A to travel from M to Y will be ‘10 - t’ minutes. We are told that train B takes 9 minutes to reach X after it meets train A, which means, train B takes 9 minutes to travel from M to X.
When two bodies travel equal distances at constant speeds, the ratio of time taken by them to travel those distances will be the same.
The time taken by A and B to travel the distance between A and M is ‘t’ minutes and 9 minutes respectively. Similarly, the time taken by them to travel the distance between M and B is ‘10 - t’ and ‘t’ minutes.
The ratio of the times taken should be equal.
t/9=(10−t)/t
=9(10−t)
=90−9t
+9t−90=0
+15t−6t−90=0
t(t+15)−6(t+15)=0
(t+15)(t−6)=0
t = - 15 or t = 6
t can’t be negative, therefore, t is 6.
The total time taken by train B to reach station X from station Y is 6 + 9 =
15 minutes.
THE ANSWER IS 15 MINUTES.
5
24/12/2023 4:09 pm
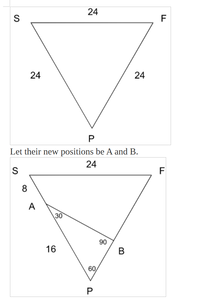
Two ships are approaching a port along straight routes at constant speeds. Initially, the two ships and the port formed an equilateral triangle with sides of length 24 km. When the slower ship travelled 8 km, the triangle formed by the new positions of the two ships and the port became right-angled. When the faster ship reaches the port, the distance, in km, between the other ship and the port will be
A. 8
B. 12
C. 6
D. 4
Neraj (TG) 26/12/2023 11:35 am
Answer :-
Let us denote the port by P,
the slower ship by S.
the faster ship by F.
Let their new positions be A and B.
Triangle APB is right-angled, We know that angle APB is 60 degrees, because triangle SFA is equilateral. The right angle must be at point B, because angle PAB is less than 60 degrees.
sin(30) = 1/2=BP/AP
BP = 0.5(AP) = 8
BF = FP - BP = 24 - 8 = 16
That mean, the faster ship is twice as fast as the slower one.
So, when the faster ship reaches the Port covering 24 km, the slower ship covers only 12 km and has remaining 12 km left to cover.
5
17/01/2024 4:06 pm
Q1. A straight road connects points A and B. Car 1 travels from A to B and Car 2 travels from B to A, both leaving at the same time. After meeting each other, they take 45 minutes and 20 minutes, respectively, to complete their journeys. If Car 1 travels at the speed of 60 km/hr, then the speed of Car 2, in km/hr, is?
A. 90
B. 80
C. 70
D. 100
4
29/01/2024 2:36 pm
Q1. Two cars travel the same distance starting at 10:00 am and 11:00 am, respectively, on the same day. They reach their common destination at the same point of time. If the first car travelled for at least 6 hours, then the highest possible value of the percentage by which the speed of the second car could exceed that of the first car is
A. 20
B. 10
C. 35
D. 25
4
03/02/2024 1:55 pm
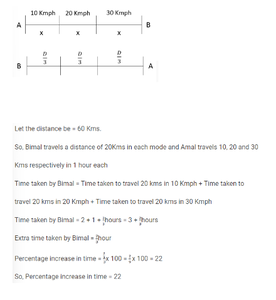
Q1. One can use three different transports which move at 10, 20, and 30 kmph, respectively. To reach from A to B, Amal took each mode of transport 1/3 of his total journey time, while Bimal took each mode of transport 1/3 of the total distance. The percentage by which Bimal's travel time exceeds Amal's travel time is nearest to ?
4
07/02/2024 12:50 pm
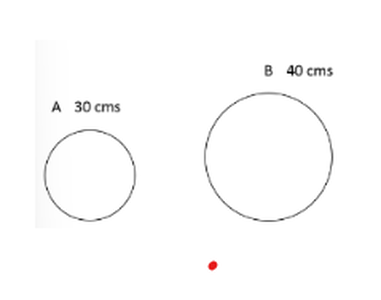
Q1. The wheels of bicycles A and B have radii 30 cm and 40 cm, respectively. While traveling a certain distance, each wheel of A required 5000 more revolutions than each wheel of B. If bicycle B traveled this distance in 45 minutes, then its speed, in km per hour, was ?
Shivangi Garg 08/02/2024 12:30 pm
@neraj-naiyar Sir,
Circumference of A and B are in the ratio 3: 4
So, Ratio of Distance travelled in one revolution by A and B = 3: 4
Since they travel the same distance,
Ratio of number of revolutions of A and B = 4: 3 ----- (1)
We know that each wheel of A requires 5000 more revolutions than B
So, the Ratio of number of revolutions of A and B = (n + 5000): n ------(2)
So, comparing (1) and (2)
Number of revolutions of A and B are 20000 and 15000 respectively
So, we know Bike B does 15000 revolutions in 45 minutes
Distance travelled = 2 x π x r x Number of revolutions
3
30/01/2024 3:47 pm
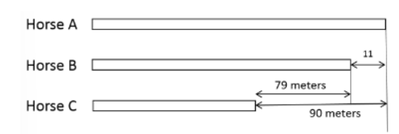
Q1. In a race of three horses, the first beat the second by 11 metres and the third by 90 metres. If the second beat the third by 80 metres, what was the length, in metres, of the racecourse?
Ujjwal Dwivedi 03/02/2024 1:12 pm
Ans – 880
A beats B by 11 meters. When B completes the 11 meters, there is a lead of 80 meters to C
So, C must have travelled only 90 - 80 = 10 meters
When B travels 11 meters, C travels only 10 meters
Ratio of distance travelled by second and third horse are11x and 10 x respectively
We know that the second horse beats the third horse by 80 meters.
So, Length of the track = Distance travelled by the second horse = 11 x 80 = 880 meters
3
20/02/2024 3:40 pm
Q1. In a 1000 meter race Rishi beats Koustab by 50 meters. In the same race, by what time margin Koustab beat Pandey who runs at 4 meter per second?
2
21/02/2024 12:30 pm
Q1. Guru and Chela walk around a circular path of 115 km in circumference, they start together from the same point. If they walk at speed of 4 and 5 kmph respectively, also they walk in the same direction, when will they meet?
A) After 115 hours
B) After 125 hours
C) After 120 hours
D) After 210 hours
Ujjwal Dwivedi 28/02/2024 5:12 pm
This post was modified 6 months ago by Ujjwal Dwivedi
They start together at same point and in same direction and in same direction.
Relative speed for both = 5 - 4 = 1km/hr
So, after end of 1 hr, Guru covered 4km
And Chela would have covered 5 km
Gap between them is 1 km/hr
Faster person has to overtake and do 1 additional round for them to meet.
Since relative speed is 1km/hr
1 additional round is the time required for meeting
So, 115/1 = 115 hours.
1
31/03/2018 3:00 pm
A motorcyclist has to cover a distance of 200 km to reach city b from city a. After travelling a certain distance, his motorcycle develops a problem and travels at 3/4th of its original speed, thereby he reached b 1 hour late. Had the problem developed 30 km earlier, he wud hav reached b 12 minutes later. Find the initial distance it travelled without a problem and the speed over that part of the journey
1
31/03/2018 3:00 pm
Difference of 12 minutes comes because of 30 km.
--> Difference of an hour comes because of 150 km.
Therefore, the problem happened after 200 - 150 = 50 km.
Let the original time taken for this 150 km track was t.
With 3/4th speed, new time will be 4t/3.
Extra time = 4t/3 - t = 1 h (given) --> t = 3h. The train takes 3h to cover 150 km. Therefore, its normal speed is 50 kmph. Speed after the problem = 3/4th of the speed = 37.5 kmph
1
09/07/2018 2:06 am
Appu and Gappu both start running around a circular racetrack in the same direction, each of them going at a constant speed. Appu is on a bicycle and Gappu is on foot. Appu goes all the way around the track and catches up with Gappu. The moment he meets Gappu, he turns around and heads back the starting point while Gappu keeps on going on his path. When Appu reaches the starting point he again meets Gappu who is just finishing his first round. What is the ratio of the speeds of Appu and Gappu?
TG Team 10/07/2018 5:21 pm
Hi Nilesh !
Let A is the starting point and B is the point where Appu and Gappu meet the first time .
Let the smaller distance between A and B is y and larger one is x .
x/ ( 2x + y ) = y/x
x^2 = 2xy + y^2
dividing the equation by y^2 ,
(x/y)^2 = 2x/y + 1
on solving we get x/y = 1 + sqrt{2} or 1 - sqrt{2}
Hence ,the ratio of speeds of appu and Gappu is 1 + sqrt{2} : 1 .
1
09/10/2018 1:49 pm
Ram starts on his Honda Civic from CL center (A) towards Workshop arena (B). Ramesh starts on foot from the same point, A towards point B at the same time. After some time, Ramesh calls up Ram, just when Ram has traveled half the distance. Ramesh continues towards B while Ram turns back. He picks up Ramesh and together they turn back again and reach B, taking 1.5 times the time Ram would normally take while Ramesh saves 15 minutes. If AB = 3 kms, the the speed of Ramesh is
1
02/12/2018 11:42 am
Page 1 / 5
Next