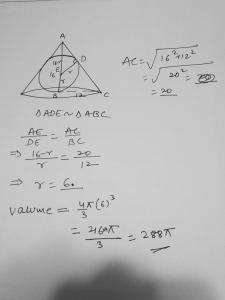04/03/2018 2:35 pm
Topic starter
Geometry
52
Posts
17
Users
4
Reactions
15.7 K
Views
2
If three sides of a rectangular park have a total length of 400 ft, then area of park is maximum when length(in ft) of its longer side is ?
Anonymous 05/03/2018 11:41 am
Let the length and breadth of the park be l and b, l > b
Case 1: 2l + b = 400
Area = lb. Area is maximum when 2l * b is maximum, which is maximum when 2l = b
=> l = 100, b = 200. Which can't happen since l > b
Case 2: l + 2b = 400
Area = lb. Area is maximum when l *2 b is
maximum, which is maximum when l = 2b
=> l = 200, b = 100.
Hence the length of the longer side is 200 ft
21 questions & discussions are there under this sub-topic
2
13/10/2018 12:58 pm
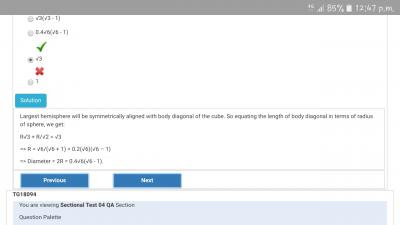
What is the diameter of largest hemisphere that can be inscribed in a cube of edge length 1?
√3(√3 - 1)
0.4√6(√6 - 1)
√3
1
aniket prajapati 13/10/2018 3:11 pm
This post was modified 6 years ago by aniket prajapati
Place a hemishphere such that ends of it's diameter coincide with opposite vertices of any face of cube
Diameter = diagonal of cube =a√3
Here a=1
So diameter of hemishphere =√3
You can check that other values are less than √3 hence option (c)
aniket prajapati 16/10/2018 11:30 pm
diameter will be greater than 1 and less than √2 hence only 2 option satisfies
aniket prajapati 16/10/2018 11:56 pm
This post was modified 6 years ago by aniket prajapati
place a bowl in a box you will notice that the bowl is tangent to 2 adjacent faces and those two points will be on the square as shown in the figure so we can conclude that it will be less than diagonal of the square which √2
this is my theory I may be wrong I tried it today and got this way I tried to make this figure
if you come up with a better solution or any strong point against it please do share in future
try it practically
0
31/03/2018 2:21 pm
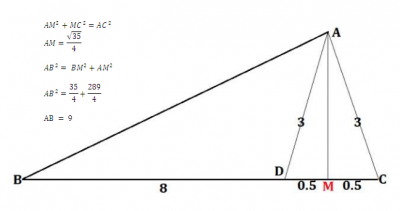
A sphere is inscribed in a cone whose radius and height are 12 and 16 units, respectively. Then, the volume of the sphere is
Choose one answer.
| a. 256pi | ||
| b. 288pi | ||
| c. 216pi | ||
| d. 312pi |
0
03/07/2018 5:38 pm
0
21/08/2018 9:30 pm
aniket prajapati 23/08/2018 10:39 pm
This post was modified 6 years ago by aniket prajapati
PFA ,
bigger cube will be converted into cube of dimension (6*6*6)
u can see one face of cube in figure those which are coloured will only be painted on one side and the cubes in edges will be coloured in more than 1 side
hence for 1 face =4*4=16
for six face =16*6=96
0
25/09/2018 12:46 pm
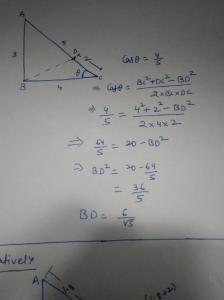
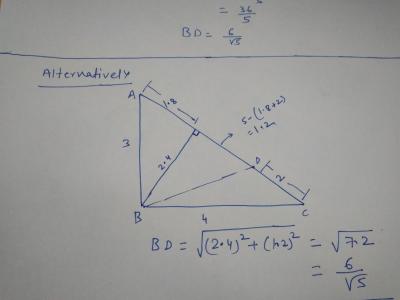
A regular tetrahedron of length 12 cm is accommodated in a sphere such that all its vertices touch the sphere. Find the radius of the sphere. Options : 1)7.2 cm 2)7 cm 3)7.5 cm 4)6.5 cm
aniket prajapati 25/09/2018 11:43 pm
in tetrahedron height h = (√6/3)a where a = length of an edge
so, in this case, h=(√6/3)*12 which is (√6)*4
we know the height of tetrahedron is divided in the ratio of 2:1 from the centre of the sphere or centroid of the tetrahedron
so radius of sphere =(2/3)*(√6)*4=8*2.44/3
=19.52/3=6.5
hence option (4) is correct
aniket prajapati 26/09/2018 12:25 pm
U can also learn directly
Radius of sphere inside tetrahedron= h/4
And radius of sphere circumscribing tetrahedron =3h/4
0
03/10/2018 12:08 am
0
17/10/2018 10:27 pm
How many of the following rectangle cannot be divided in two equal parts which can be rearranged to form a square?
16×9
18×8
24×6
36×4
0
17/10/2018 10:30 pm
Find the number of different shortest paths from point A to point B in a city with perfectly horizontal streets and vertical avenues as shown in figure. No path can cross the fenced off area shown in grey in the figure.
16
17
19
0
05/11/2018 12:35 pm
The height of a trapezoid whose diagonals are mutually perpendicular is equal to 4. Find the area of the trapezoid if it is known that the length of one of its diagonal is equal to 5.
ans: 50/3
0
12/11/2018 8:47 am
The parallel sides of a trapezium are of 6 units and 8 units respectively. Find the length of the line segment parallel to these sides and which divides the area of the trapezium in equal halves.
4√3
5√2
0
12/11/2018 8:48 am
ABCD is a square. P and Q are points on the sides, as shown, such that BP: PC = 3: 1 and BQ: AQ = 2: 1. Find the ratio of the area of the shaded region to the area of the square ABCD.
5/12
7/24
0
13/11/2018 6:58 pm
Sir , kindly share the solution to this problem -
0
14/11/2018 8:45 pm
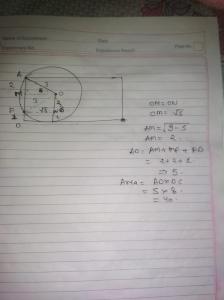
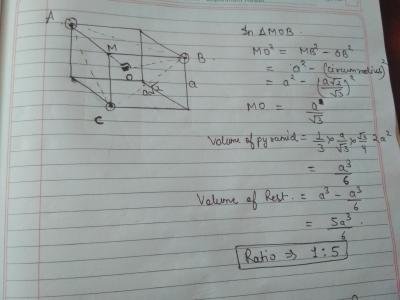
A cube is divided into two parts by a plane passing through three non-adjacent vertices. Find the ratio of volume of the smaller part to that of larger one.
Ans: 1:5
0
15/11/2018 10:57 pm
Sir , please share the solutions of questions 25 and 26 -
TG.Raman 15/11/2018 11:32 pm
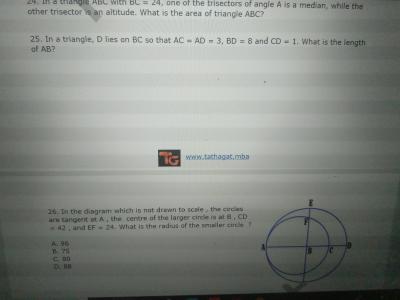
26.
Let the larger radius be x. By the power of a point theorem,
AB BC = FB2
x(x-42) = (x-24)2
6x = 242,
x = 96.
Now the smaller radius is AC/2 = (AB+BC)/2 = (2x -42)/2 = x-21 = 75. Hence the radius of the smaller circle = 75.
Page 1 / 2
Next